
The management of spare parts and inventories is all about balance between the cost of stocking the parts and the costs and risks of not having them when they are required.
As a consultancy owner, one of the most overlooked issues I see in Industrial operations is the management of spare parts. I don’t have enough fingers, toes and limbs to count how many times I’ve heard the phrase ” The machine is down because we’re waiting on spare parts”.
I see this phrase normalised so often that people don’t bat an eye and have accepted it as the norm- a lot of maintenance professionals don’t focus on the effective management of this area . I think this is overlooked because it requires MATH to calculate- and just like poetry, most people hate math.
Luckily, doing Math is how I make a living, and because us Engineers have a pretty open community of sharing information, I thought I’d write this article that may help a few of you at your operations.
Introduction
The management of spare parts and inventories is all about balance between the cost of stocking the parts and the costs and risks of not having them when they are required.
Spares management includes areas such as:
- Parts forecasting
- Deciding what to stock and provisioning appropriately
- Identifying what is stocked and where it is held
- Arranging safe handling pf parts
- Recording movements of inventories, in/out and balances
- Controlling misuse or theft
- Minimising obsolescence
Costs Associated with Spares and Repairable Items
Companies keep spare parts to be able to replace worn out or defective parts in machinery and equipment. Typically Mining companies hold parts that run into the tens of millions of dollars.
With Mines located in rural areas where access to dealerships is limited, this inventory amount will increase drastically as long lead times are needed to order, dispatch and transport parts from overseas or major towns. The failure of a critical component in service at the mine may result in an unacceptable downtime cost should a spare part not be available at site.
In the management of spare parts and inventories, a balance is sought between the cost of holding the part in stock, versus the cot and risks of not having them when they are needed. Holding excess inventory ties up funds that could be more usefully employed in other activities. This opportunity cost of stockholding is the difference between the best alternative investment and the return from the spares holding (Lawrenson, 1999).
In a simple sense, we aim to minimise the total cost of:
- Part acquisition (or “Ordering”) costs
- Inventory holding costs
- Stock-out costs
It is to be noted that spares aren’t always kept for financial reasons. There may also be safety and environmental, or strategic reasons for carrying spares. Defence applications where it is necessary to maintain a given level of deterrent capability is a good example of keeping strategic spares.
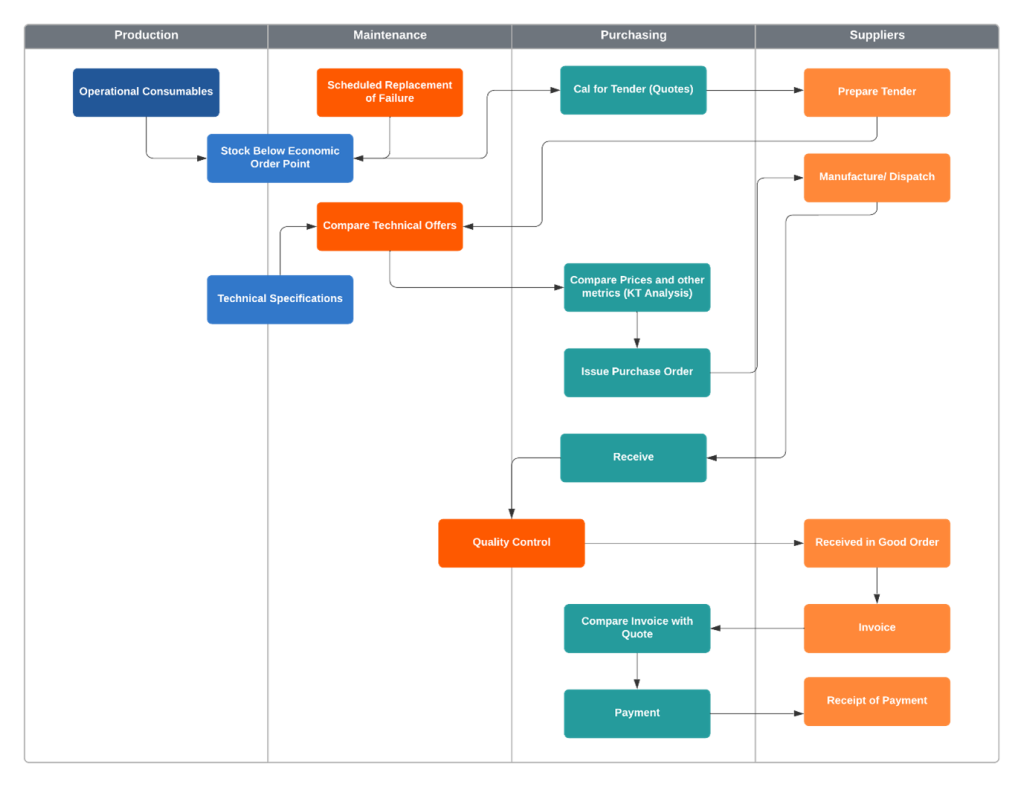
Figure 1 shows the simplified process of acquiring spare parts.
This process requires interaction between Operations, Maintenance, Purchasing and the Supplier. The decision to purchase inventory is triggered when the level of spares or consumables falls below a certain point, known as the Economic Order Point.
In some cases where suppliers have previously submitted to a pre-qualification process, a Purchase Order might be automatically issued. If the spare is available from the OEM and alternative sources, a request for quotation will be issued to the competing vendors. Offers will firstly be compared on the basis of satisfying fitness for duty criteria, and safety & environmental compliance. The supplier with the winning tender is formally notified, and a PO issued. The spare is dispatched, received and inspected by the warehouse. As can be seen from Table 1, this can be a labour-intensive process. Even though the process includes multiple steps, spare parts literature often refers to the associated process cost as the “Ordering Cost”.
Spares holding costs are frequently expressed as a percentage of capital cost of inventory. They include:
- The annual stockholding cost. Less expensive spares are purchased using OPEX, while high value items are usually out of CAPEX. (Depends on the use for accounting purposes). The repayments on this capital (compromising of both interest and amortization) form part of the annual stockholding cost at between 15-19% of inventory value
- The cost of Obsolescence. This considers the decline in market value of spares due to new and improved components entering the market. It is analogous to the decline in the resale value of a car once a new one is introduced. On high-value items the depreciation rate can be used to estimate the cost of obsolescence.
- Warehouse Operating Costs. These include labour, insurance, database admin, and warehouse services. Some components have associated warehouse inspection and maintenance costs- e.g. electric motor shafts should be rotated on a periodic basis to avoid flattening of the bearing elements.
When these cost estimates are combines, spares holding costs can be estimated at between 38-48% of the capital value of spares. This implies significant operating costs for maintaining high dollar value inventories.
Stock-out costs are associated with loss of product of quality. Product and quality losses are incurred through losses of overall equipment effectiveness, either via availability losses, throughput limitations or declining yields in processing plants.
Spares Classification
Non-repairable items are discarded following completion of a single usage cycle. It is not economic to transport and/or repair or recondition these components. Examples of non-repairable components include oil filters, light bulbs and Ground Engaging Tools (GET).
Repairable items such as diesel engines, wheel motors and pumps are also referred to as “Rotables”. They undergo successive cycles of usage and repair. Often, repairs are conducted off-site due to the specialised nature of skills required. Repairable items are made up of sub-assemblies of components. Components fail in different ways, by wear-out, fatigue, random failure or infant mortality. If components fail by wear, they will experience different wear rates. It is clearly uneconomical to repair or replace all components of each subassembly.
The fundamental question to ask during repair includes: “Which sub-assemblies and components need replacement or repair?”.
There is an economic imperative to minimise repair costs subject to the risk of the components failing prior to warranty when it is returned to service. Repairs can sometimes be carried out at site or at a centralised depot. Such systems are called multi-echelon systems (Figure 3).
Figure 3: Multi-echelon repair system (Louit, 2007)
Both non-repairable and repairable spares are further categorized as high or low rotation, based on their annual dollar usage-to-stock ratio. Non-repairable items vary in unit value, while low-rotation repairable spares, like motors, transmissions, and final drive wheel groups, are typically high-value units. Re-order quantities and points are determined by the spare’s rotation frequency, dollar value, and criticality.
Spares Demand and Service Level
It is important to understand that when determining spares levels there are two key areas:
Spares determined by Operational Demand and Equipment Reliability
- This can be further split into two categories, namely parts required for Preventative Maintenance (PM) or otherwise knows as scheduled maintenance, and those required for Corrective Maintenance (Unplanned). PM Spares are relatively straightforward to calculate, however CM spares require a bit of failure risk modelling and selection of a desired part availability
- This process is mainly used for Low-Rotation Critical Spares such as conveyor motors, Final Drives, Cylinders etc.
Spares level determined by Supplier lead time, holding cost and Demand variability
- The spares quantity determined in item one is the required spares that should be on hand at any given moment if the required part availability is to be met.
- If the lead time on a part is large, the stores might opt to increase this holding to ensure the required qty is always on hand.
- For High Rotation items, trends and distributions of Demand can be used instead of the reliability requirements, and the stock level calculations can be performed using the EOQ or EOP method covered further in the document.
From the above, an example would be if the Mine modelled the failures of the Hoist cylinders on a fleet of Haul trucks and determined on a basis of combined Spares required for both planned and unplanned maintenance, that there should be 10 cylinders in stock to ensure there is a 99% chance of the required part being available when needed.
The stores would then take this operational requirement, and further model with the lead time and demand and service level, the required ordering amount and economic order point.
High Rotation Spares
Constant Demand, Zero Lead-Time
One of the main problems in spares management is knowing the quantity to order considering the demand and lead time. The following items draw heavily from Lawrenson, 1986.
Figure 5: Stock Variations over time (Lawrenson, 1986)
Figure 5 shows a simple model of the variation of stock over time for an individual stock item. The model assumes constant demand and instantaneous reprovisioning (i.e. zero lead-time). It can be seen that, if the warehouse orders batches of Q parts, and reorders when the parts are exhausted, the average number of components held in stock is Q/2. The annual stockholding cost is therefore:
\[Cost=\ P\times I\times\frac{Q}{2}\]
Where P is the price of the components, and I is the holding factor associated with the holding costs of the component (expressed as a percentage). Thus, the cost is proportional to Q.
If the annual demand for components is D, then the number of orders made per year will be D/Q. If we assume that there is a constant cost, C, associated with placing an order, then the order costs are inversely proportional to the order quantity, Q, and we can write:
\[Cost=\ C\times\frac{D}{Q}\]
Figure 6: Total Stockholding and Ordering Costs (Plossl, 1985)
The total cost associated with ordering and holding spares is given by summing the two previous equations. The minimum cost is given by differentiating the equation. It can be shown that this is the equivalent of finding the point of intersection of the two cost component curves, thus:
\[P\times I\times\frac{Q}{2}=\ C\times\frac{D}{Q}\]
By rearrangement we find that the optimum batch size, Q, or Economic Order Quantity (EOQ) is given by:
\[EOQ=\sqrt{\frac{2DC}{PI}}\]
Where:
- D= Demand Items per year
- C= Cost of ordering a line item
- P= Item Price
- I= Stockholding rate (Per annum percentage)
This is known as Wilson’s formula.
Example:
- D= 120 items per year
- C= $20 per order
- P= $100 each
- I= 20% per year
\[EOQ=\sqrt{\frac{2\times120\times20}{100\times0.2}}=15.49\approx16\ Spares\]
Constant Demand, Constant Lead-Time
In the case that the lead-time necessary to re-order spares is constant, we can define a trigger point as the minimum re-order level.
The re-order level R, can be calculated by:
\[R=D\ \times T_L\]
Where:
- D= Monthly demand
- = Lead Time (in months)
Variable Demand, Constant Lead-Time
Demand for spares is rarely constant. If we suppose that demand follows a normal distribution (Figure 8), then the mean demand curve can be represented by D and the spread of demand is determined by the standard deviation, .
With a mean demand of 50 items per month, and a standard deviation of 5 items per month, we can be 99.9% certain that the demand will lie between plus/minus three standard deviations, or 50 +/- 15 items. Thus, we could define a safety stock of 15 items.
IF we suppose that there is a two-month lead time to obtain spares, then the demand for the spares may be high in month one, and low in month two. We would therefore not define the number of safety spares as 2 x 15 =30 components. In general, the rule is to increase the proportion by the square root of the lead time. Thus, an adequate number of safety stock is Spares.
In general, the Economic Order Point (EOP) is given by:
\[EOP=\ (D\times T_L)+\ (k\ \times S_D\times\sqrt{T_L})\]
Where k is a safety stock factor determined by the required service level for the component. It is the z-score for a normal distribution.
Example:
Suppose the demand for oil filters varies between 36 and 54 per month with a lead time of 2 months when an order is placed. If we assume that the demand is normally distributed, then the average demand is (36+54)/2= 45 units.
3 x = 9, so the standard deviation is thus 9/3= 3.
For a 99% service level, the EOP is thus:
\[EOP=\ (D\times T_L)+\ (k\ \times S_D\times\sqrt{T_L})=45\times2+2.33\times3\times\sqrt2=99.9\approx100\ Units\]
CASE STUDY
Calculating Required Spares Holding (Operational Demand for Low-Rotation Spares)
A Mine has determined that the optimal maintenance interval for cylinder replacements are at 3,500 hours. The Reliability Engineer passes on the calculated Reliability Metrics from a Weibull Analysis, which are given in the table below.
You are asked to calculate the required quantity of spares that must always be on hand to ensure a 99% level of availability.
| Component | Beta | Eta |
| Cylinder | 2.0303 | 7848 |
| Metric | Value | Unit |
| Required Availability | 0.99 | |
| Annual Hours (Max) | 8760 | hrs |
| Number of systems | 1 | (independent) |
| PM Interval | 3500 | hrs |
The failure rate formula from a Weibull distribution with shape and scale parameters is given below:
\[F\left(t\right)=1-e^{-\left(\frac{t}{\eta}\right)^\beta}\]
Using this formula we can calculate the expected failures (or CM Failures) that could occur between 0 hours and our Preventative Maintenance (PM) interval. Since the Beta value is greater than one, which indicates wear-out failure, we can expect the unplanned failures to also increase with PM time.
See Table 1 below for the calculations:
| PM Interval | Lambda(t) |
| 0 | 0 |
| 250 | 7.42384E-06 |
| 500 | 1.51628E-05 |
| 750 | 2.30254E-05 |
| 1000 | 3.09693E-05 |
| 1250 | 3.89742E-05 |
| 1500 | 4.70281E-05 |
| 1750 | 5.5123E-05 |
| 2000 | 6.32532E-05 |
| 2250 | 7.14142E-05 |
| 2500 | 7.96029E-05 |
| 2750 | 8.78164E-05 |
| 3000 | 9.60526E-05 |
| 3250 | 0.00010431 |
| 3500 | 0.000112586 |
Using the failure rate, we multiple this by the 3500 PM interval to get 0.39. This means that statistically speaking we can expect 0.39 unplanned failures before we perform planned maintenance, and we must stock spares appropriately.
Instead of just rounding this to 1 spare for CM, we use the Poisson distribution to model the required CM spares.
This is because the actual number of failures during the PM interval may vary due to the random nature of failures, and the distribution allows us to select an number of spares for certain confidence level.
The average CM failure we calculated (0.39) will be used as our Poisson distribution’s starting point.
The Poisson Distribution formula is given by:
\[P\left(X=x\right)=\frac{\lambda^xe^{-\lambda}}{x!}\]
Since we require the cumulative value of the Poisson formula, we make an amendment to the above to get:
\[P\left(X\le y\right)=\sum_{x=0}^{y}\frac{\lambda^xe^{-\lambda}}{x!}\]
| No. Spares | Poisson Distribution |
| 0 | 0.674319699 |
| 1 | 0.940036017 |
| 2 | 0.9923889 |
| 3 | 0.999265468 |
| 4 | 0.999942898 |
| 5 | 0.999996286 |
| 6 | 0.999999792 |
| 7 | 0.99999999 |
| 8 | 1 |
| 9 | 1 |
| 10 | 1 |
The find the final number of CM spares required, we select the No of spares that corresponds to the Poisson Distribution cumulative number equal to our part availability requirement (99%)., which in this case comes out to two spares.
It can also been seen from the table and Figure 9 below that the rate of spares required for an increase in part availability declines with an increase in qty, thus consideration should be given to the actual required part availability, as the stockholding cost can easily balloon.
The final spares calculation is shown in Table 3 below. It can been seen that rounding wasn’t performed until the very end, as to minimise over-allocation of spares and cost.
| Spares Requirements | |
| PM Parts required | 2.502857 |
| Avg CM Parts Required | 0.394051 |
| CM Spares Required (99% Availability) | 2 |
| Total Spares Required | 5 |
I’ve consolidated the above information in a succinct PDF below:
If you are interested in more of my articles and consulting services, please see my Accendo Profile and Website at : pardusconsulting.com
Miguel Pengel










Leave a Reply